Well Inflow Analyses
The well's productivity index (PI or J is common) is:
![]()
Under pseudo-steady state (pss), a calculated PI is:
![]()
The effective permeability-thickness in md-ft (![]() )
and skin (s) are estimated based on well test analyses. The external
radius,
)
and skin (s) are estimated based on well test analyses. The external
radius, ![]() ,
is the radius in feet to the no-flow boundary, based on the approximate
drainage area of the well. The radial flow equation assumes a bounded
circular drainage area, a fully penetrating well, the well is vertical
and flow is single phase. This is in addition to the assumed conditions
in Darcy's law.
,
is the radius in feet to the no-flow boundary, based on the approximate
drainage area of the well. The radial flow equation assumes a bounded
circular drainage area, a fully penetrating well, the well is vertical
and flow is single phase. This is in addition to the assumed conditions
in Darcy's law.
A zero skin damage PI (or ideal PI) would be:
![]()
Presentation of pressure transient test results for developed fields should include the history of PI's. A time plot showing PI's and idealized PI's (or rates and ideal rates) for a particular well and surrounding area, should be part of reservoir monitoring to identify common problems.
A positive skin factor indicates there is a pressure decline in the near
vicinity of well that is more than expected based on the radial flow equation.
A positive skin factor does not necessarily indicated formation damage.
However, as a conceptual model, if we consider the reservoir has been
damaged out to a radius, ![]() ,
then the skin of this zone can be calculated based on the reduced permeability:
,
then the skin of this zone can be calculated based on the reduced permeability:
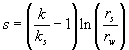
The difficulty in the above equation is knowing the damage radius. From the calculation of skin, the additional pressure drop due to skin is:

Multiphase PI
The specific PI per foot of
producing interval, or:![]() allows comparing wells with different producing intervals. Also, the steady-state
radial flow equation has been used by some authors:
allows comparing wells with different producing intervals. Also, the steady-state
radial flow equation has been used by some authors:
![]()
While technically incorrect, the effect of disregarding the -3/4 may
be insignificant. The PI as calculated with the pseudo-steady equation
or with the steady state equation, may consider multi-phase flow, with
each flow converted into reservoir barrels by its respective rate. The
multiphase flow equation substitutes for ![]() and
and
![]() , the total
transmissibilities and reservoir withdrawals as:
, the total
transmissibilities and reservoir withdrawals as:
![]()
![]()
The constant of 5.615 is used to convert cubic feet to barrels.
Note that the PI as determined from production tests may also be calculated based on total volumes of produced water, oil and gas, all stated in terms of stock tank barrels.
Flow Efficiency
Flow efficiency is the actual PI/( theoretical PI without skin). In terms of drawdown, it is:
![]()
Flow efficiency for two flow tests will be included later in this discussion.
Reservoir boundaries
Pseudo- steady pressure declines in irregularly shaped reservoirs have been determined using superposition of the transient flow equation:
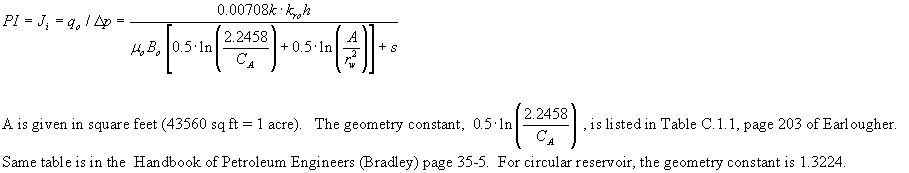
The Pseudo-Steady State Period
It is convenient to subdivide these flow periods (or shut-in periods) into 3 regions: early time, middle time and late time. Early time is affect by well bore storage. The middle time is the semi-log straight line pressure response on the drawdown test. Late time is affected by well boundaries, and this is when the pressure response transitions from infinite acting to pseudo-steady states.
The time to the start of pseudo-steady state is obtained from the shape
factor table, under the column, "exact for ![]() "
which can be converted into time in hours by:
"
which can be converted into time in hours by:
![]()
. The start of pseudo-steady state is when the farthest boundary has been felt at the wellbore. A reservoir that has transitioned to pseudo-steady state will have a linear decline in pressure with constant production. The approximate and the exact times to reach pseudo-state, from the shape factor chart, for a circle are fairly close (0.06 and 0.10). However, in cases where the well is positioned much closer to one side, the time from "approximate beginning" and "exact beginning" is much longer. This can also be seen in the plots of dimensionless pressure verses time.
The pseudo-steady state period can, at least in theory, provide estimates of the reservoir pore volume:
![]()
Empirical Well Inflow Equations
Vogel's Inflow Curve
Well inflow equations do not account for the phase change in solution gas reservoirs. In a solution gas drive, there is expansion of the hydrocarbons below bubble point, which is beneficial because it adds energy to the system. Gas liberation in a solution-gas drive is also detrimental to oil production because it lowers the effective permeability of oil.
Vogel's curve is entirely empirical, based on a series of single well solution-gas simulation runs, with varying oil properties
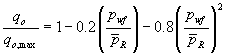
The Vogel curve, will provide the maximum rate possible given the test's rate, reservoir pressure and flowing bottomhole pressure.
Future additions -- Partial completions, gas well inflow relationships, isochronal tests.
References:
1. Wattenbarger, R. A., Well Performance Equations, Chapter 35, Petroleum Engineering Handbook (Bradley), 1987.
2. Lee et al, Pressure Transient Testing, pages 265 to 266 for shape factors, Pseudo-steady state equation, page 15. pore volume calculation, page 15, equation 1.162b. Note additional problems on skin damage on page 16. 2003.
3. Brown, K and Beggs, D, The Technology of Artificial Lift, Chapter 1, Inflow Performance, page 13 for Vogel discussion.1977.
4. Earlougher, R.C, Advances in Well Test Analysis, 1977.